Services on Demand
Journal
Article
Indicators
-
 Cited by SciELO
Cited by SciELO -
 Access statistics
Access statistics
Related links
-
 Similars in
SciELO
Similars in
SciELO  uBio
uBio
Share
Revista de Biología Tropical
On-line version ISSN 0034-7744Print version ISSN 0034-7744
Rev. biol. trop vol.54 n.2 San José Jun. 2006
Age-growth models for tilapia Oreochromis aureus (Perciformes, Cichlidae) of the Infiernillo reservoir, Mexico and reproductive behaviour
Lourdes Jiménez-Badillo
Centro de Ecología y Pesquerías. Dirección General de Investigaciones. Universidad Veracruzana. Calle Hidalgo 617. Col. Río Jamapa. Boca del Río,Veracruz, México. C.P. 94290. Phone: 52 (229) 9-56-70-70. Fax: 52 (229) 9-56-70-70; ljimenez@uv.mx
Received 19-II-2002. Corrected 16-XI-2004. Accepted 08-II-2006.
Abstract. In presence of a decrease in the catch from Infiernillo reservoir, Michoacán-Guerrero, México, age and growth of tilapia Oreochromis aureus (Steindachner 1864) were estimated, as indispensable attributes for fishery management. Evaluations included scales and opercular bones readings and length frequency analysis. The population structure was determined too. Due to the great variation of tilapia individual growth and with the objective to have reliable growth estimations, linearized, non-parametric and non-linear methods were used and their applicability discussed. Comparison of ages determined from these methods revealed that opercular bones readings had advantages over scales. Reproduction and competition for space and food were considered as responsible factors for ring-formation on tilapia hard structures. The non-linear method was considered the best for modelling populations highly variable, which is characteristic of tilapia and tropical fish with multiple spawns. The growth parameters estimated were L∞ = 479 mm, K = 0.46/yr, to = -0.055 yr. The tilapia population in Infiernillo reservoir is dominated by juveniles less than 1.5 years old. The fishery activity is affecting the tilapia population growth because are caught when they have not yet spawned. Therefore, this fishery is in urgent need of regulation. Increasing the mesh size of the gill nets might contribute to protect the reproductive potential of the stock. Rev. Biol. Trop. 54(2): 577-588. Epub 2006 Jun 01.
Key words: age growth models, reproductive behaviour, Oreochromis aureus, scales, opercular bones.
Tilapia, which is a tropical fish introduced around the world, has offered economical and social benefits mainly for rural communities, where it is caught and cultured. It constitutes a cheap and nutritious food and a source of income and employment. In México, this fishery supports more than 44 000 inhabitants of Michoacán and Guerrero states (Anonymous 1998). The highest volumes of tilapia in the country are catches from the Infiernillo reservoir (40 000 hectares). In 1987, this reservoir was the highest producer of tilapia in Latin America, with a landing of 18 953 tons (Juárez 1995). Recently, the catch has decreased to 4 770 tons (Anonymous 2000).
In presence of this situation an ecological and fishery evaluation was conducted to understand the decreases in the captures and generate basic information to propose a regulation of the fishery. Estimates of fish growth rates and age structure data are indispensable attributes of fish stocks that should be known for fisheries management. So, this research was conducted because of the necessity of knowing tilapia growth rates and age structure of the population as basic information for the stock management.
In comparison with other fish, tilapia experiences highly variable growth caused by several extrinsic and intrinsic factors such as environmental temperature, food available, metabolic activity, reproductive activity, pool genetic, etc, which produce differenced budget of catabolism and anabolism reflected in L∞ and K parameters (Isaac 1990).
For tilapia, the multiple reproductive periods constitutes a relevant aspect in their life. Energy expenditure is very intense during spawning periods and has an important effect on growth (Babiker and Ibrahim 1979). In tilapia population is common to observe mixed cohorts as consequence of multiple spawning. Also longer fish of short age and small fish of old age are often observed. Such variability produces difficulties to obtained reliable growth estimations. In these cases linearized models fail to describe the growth adequately, so one non-linear model could be used as a better alternative. Methods such as those based on length frequency analysis, broadly recommended for tropical fish growth evaluations (Sparre and Venema 1995) were also used in this case.
Materials and methods
Infiernillo reservoir is located in Michoacan-Guerrero, Mexico at 18º1630 N and 101º5340 W. Biological sampling -consisted of trawls with beach seines 50 m long and 1 cm mesh- and samples of commercial capture -caught with gill nets 8.3 cm and 10.8 cm meshwere obtained in the main fishing localities, in order to have a representative sample of all tilapia life stages. The sample sizes were determined according to Eckblad (1991).
For each fish in the total sample, the standard length (SL), total weight (W) and sex were recorded. The length-weight relationship for individuals of each sex was computed according to Ricker (1975). To determine growth differences between sexes, statistical tests for comparing two slopes and two intercepts were made according to Zar (1974). Length frequency distributions were obtained using 5 mm class intervals according to Wolff (1989), to estimate growth by indirect methods.
The number of individuals on which readings of scales and opercular bones should be made was determined using proportional stratified sampling (Castro and Lawing 1995). So, the scales and opercular bones from a random subsample of 400 individuals were extracted, and the stage of maturation of these fish was recorded. The hard structures were stored, washed, treated and mounted according to FAO (1982). The number of growth rings on three scales –for the high variability- and both opercular bones on each fish, were counted. The focus-to-ring distance of each growth ring was measured on two separate occasions to corroborate them following FAO (1982). To interpret growth rings on hard structures, three criteria were used: 1) marginal increment analysis; 2) frequency distribution analysis of focus-to-ring distances for different age groups and 3) comparison of age groups obtained through scale and opercular bone readings and cohort estimates from length frequency analysis. The first criteria established the time and period of growth ring formation. The second revealed the occurrence of a mode for each ring and its consistency between different ages. The third corroborated age groups from indirect and direct estimates.
The relationship between average distances of growth rings on both hard structures and the fish length was computed, and then used to establish growth rates (Bagenal and Tesch 1978). The relationship between tilapia growth ring formation on hard structures and reproductive activity, feeding intensity and fluctuating water level of Infiernillo reservoir were analysed, superimposing tendencies.
A length-age key was developed from scales and opercular bone readings. From this, length frequency distributions were transformed into age frequency distributions according to Ricker (1975), in order to establish the age structure of the tilapia population in Infiernillo reservoir.
The growth parameters for tilapia were obtained after assigning an age to each of the average fish lengths and based on Walford (1946), Beverton and Holt (1957), Gulland and Holt (1959), Bayley (1977), Munro (1982) and non-linear methods (Saila et al. 1988). The indirect methods used to estimate growth parameters from length frequency distributions were SLCA (Shepherds Length Composition Analysis), PROJMAT (Projection Matriz Method) and ELEFAN (Electronic Length Frequency Analysis), which are non-parametric methods included in the LFDA package (Length Frequency Data Analysis; Jones et al. 1990). Bhattacharya, Modal Progression and Gulland and Holt analyses included in FISAT package (FAO ICLARM Stock Assessment Tools; Gayanilo et al. 1993) were also used.
The growth parameters that reliably describe tilapia growth were selected considering the best fit of the model with the observed data, measured through a score. The score for the ELEFAN and the SLCA methods corresponds to the maximum value of ESP/ASP (Explained Sum of Peaks / Available Sum of Peaks) ratio and Smax, respectively. For PROJMAT and the methods based on hard structures, the score corresponds to the minimum value of SSD (Sum of Squares Differences).
The growth parameters obtained in this study and the estimates carried out by other authors in other reservoirs, were compared through the criteria ∅established by Munro and Pauly (1983), which compare L∞, K and to tendencies.
Finally, fishing implications on tilapia growth were analyzed.
Results
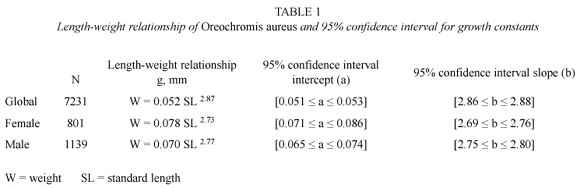
The population studied consisted of 7 231 individuals from 15 to 305 mm standard length (SL), and 0.6 to 1403.7 g total weight. Lengthweight relationships (Table 1), indicated no significant differences between sexes (two-means test, p=0.078 for slope and p=0.06 for intercept, df = 7 229, n = 7 231), therefore males and females were grouped for other analyses. The slope was significantly different from 3 (onemean test p=0.0001), which is indicative of alometric growth.
Concentric rings were observed in most scales and opercular bones. Most scales were found to be interpretable, although rings were more clearly defined in opercular bones than in scales. Scales from individuals longer than 250 mm, were not readable, due to the limited visual scope of the microscope used. The opercular bones from individuals smaller than 128 mm were not collected. Only the reliable scales and opercular bone readings were used in further analyses.
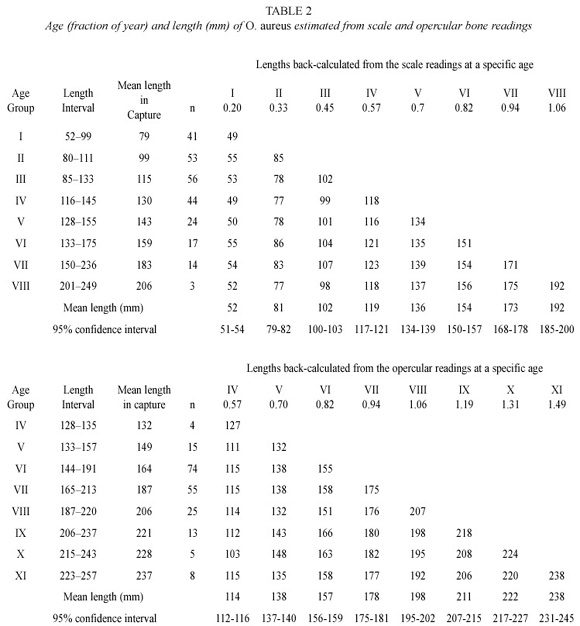
The linear relationships between focusto- ring distances on structures and fish length, were SL = -6.08 + 45.47 RS mm and SL = 18.3 + 6.11 OL mm, where SL is fish standard length, RS average radius of scales and OL is opercular length. The opercular length measured vertically was better correlated with fish standard length than when measured horizontally or diagonally.
The frequency distributions of focus-toring distances in scales and opercular bones were separated into eight components, suggesting eight age groups. Among age groups IV-VIII, where aging from both scales and opercular bones was available, age to length relation was similar. Considering scale and opercular bone readings together, 11 age groups were detected (Table 2).
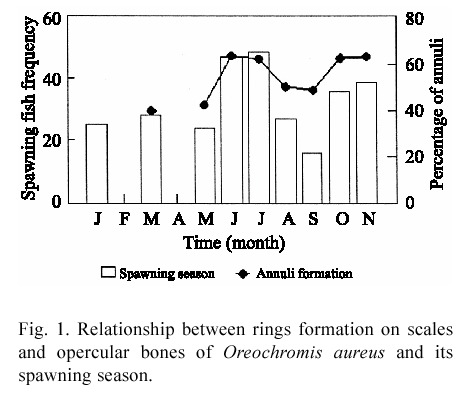
Marginal increment analysis indicated a higher percentage of rings formed on both structures margins in June, July, October and November, coinciding with tilapia spawning season (Fig. 1), which occurs from May to January, with peaks in June-July and October- November (Jiménez 1999). Apparently the periodicity between rings is around 45 days, because in August and September 54% of individuals also show rings over hard structure margins.
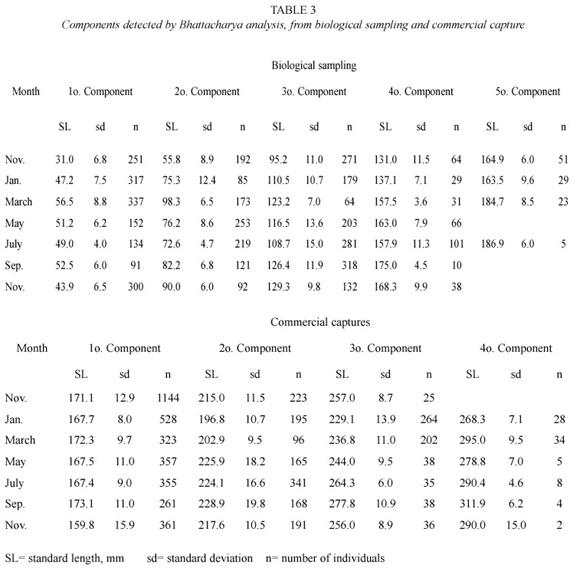
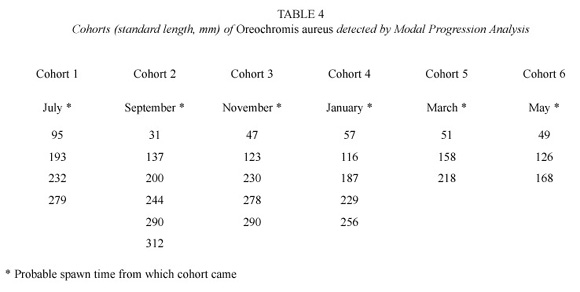
The Bhattacharya Analysis from length frequency gave the components shown in Table 3. The last component of biological sampling seems to coincide with the first component of commercial capture. The Modal Progression Analysis produced six cohorts (Table 4), whose probable hatch date was inferred extrapolating toward the axis that represents the relative age.
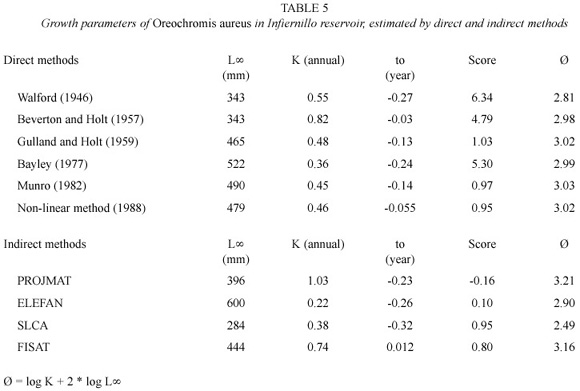
The tilapia growth parameters estimated by different methods are presented in Table 5. The Walford, Beverton and Holt and SLCA methods underestimated L∞, contrary to ELEFAN, which overestimated it.
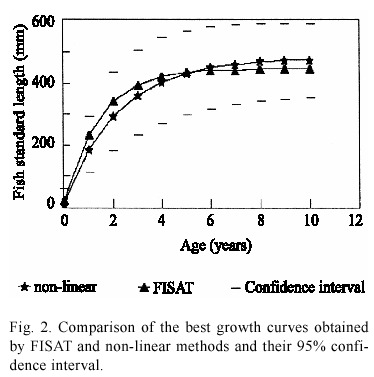
The growth curves which appeared to be more robust were obtained by non-linear and FISAT methods (Fig. 2) direct and indirect method respectively. The tendency of both curves is similar, although the parameter values differ, this confirm the estimations. Both curves are within a 95% confidence interval estimated by the non-linear method. This method was selected to describe the tilapia growth, because it provides the best fit and interval estimates for the growth parameters.
The functions that describe tilapia growth were Lt = 478 [1-e-0.46(t+0.055)] expressed in standard length (mm) and Wt = 3446 [1-e-0.46(t+0.055)]2.87 expressed in total weight (g). The 95% confidence interval for growth parameters were [336 ≤ L∞≤ 621], [0.26 ≤ K ≤ 0.66] and [- 0.11 ≤ to ≤ 0.005]. These functions indicate that the major growth of tilapia occurs in its first year of life, when on average it reaches a length of 184 mm and a weight of 241.7 g. In the second year it averages 293 mm and 877.7 g. At three years its average size increases to 361 mm and 1587.2 g. From then on, growth is notably reduced, so that by four years, average SL is 404 mm and average weight is 2182.6 g. Asymptotic growth is reached earlier than year eight (Fig. 2).
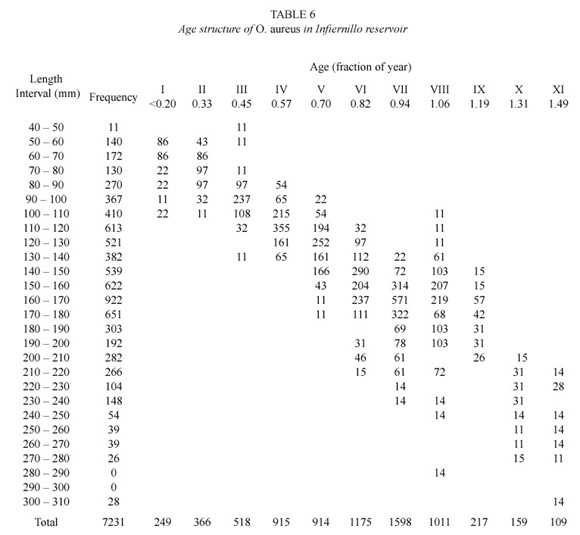
According with the tilapia age structure derived from the length-age key (Table 6), the tilapia population in Infiernillo reservoir is dominated by juveniles less than 1.5 years old.
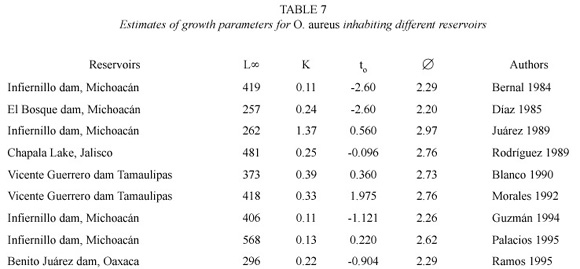
Different growth estimates for O. aureus made by different authors are presented in Table 7. The great variation in the parameters is remarkable. The L∞fluctuates between 257 to 568 mm and K between 0.11 to 1.37.
Discussion
Age and causal factors of ring formation on hard structures. Tilapias as tropical fish, faces some difficulties to be aged. First difficulty was related with the hard structures used to aged and the other to discern between factors responsible of ring formations and to determine the periodicity between rings.
In this study, twenty five percent of scales were discarded from the original sample, because they showed continuous ring on the posterior part, but crossed in the anterior part, suggesting they were false rings. In scales and opercular bones, the rings for early ages in adult fish were difficult to recognize. This suggests that carbonated material superimposed on the rings until the first ones disappeared. So, only reliable structures were used to estimate age.
The great dispersion in the scales size corresponding to a certain fish length, mainly among individuals of 110-180 mm, reflected differential rates of growth as consequence of tilapia multiple spawns and the considerable mixes of cohorts, in addition to individual variability in growth. In opercular bones, that variation was less evident and the relationship between opercular length and fish length was best fited. Since this function leads to obtain more precise estimates when it was used in back-calculation, opercular bones represented a more appropriate structure to evaluate tilapia growth. Moreover, the extraction, preparation and reading of opercular bones in comparison with scales were relatively easier.
From all factors considered to analyse the cause of growth ring formation on hard structures, only the spawning season, shown a relationship when the tendencies were superimposing. Tilapia limited feeding during incubation and brood guarding; contribute to reductions in growth rate. Also the increased demand by gonads for energy and calcium causes resorption from the scales, leaving a mark on the scale. Garrod 1959, Fagade 1973, Payne & Collinson 1983, Morales 1991 and Guzmán 1994 coincide with this assumption. So, reproductive period was considered a main factor of growth ring formation on tilapia hard structures.
Tilapias have asynchronous spawning, where ovaries do not mature at the same time, but in stages. Therefore, the egg liberation takes place sequentially for the approximately four months that the spawning period lasts. Since tilapia reproductive events occur yearround in multiple spawning, more than one ring per year could be formed on scales and opercular bones. Considering the time invested by tilapia in fertilisation, oral incubation, brood care and preparation for the following spawn, each spawning event could take place every 45 days. This periodicity causes in turn reduced growth rate and, consequently, ring formation on hard structures.
The existence of at least six cohorts per year identified through the growth indirect evaluation and the presence of around eight modal lengths per month supported the idea of multiple spawning with that periodicity. Under that consideration, cohorts 1, 2 and 6 probably came from the May to September spawns, the cohorts 3 and 4 from October to January spawns and cohort 5 from February to April spawns. Approximately two months separated each offspring; this may correspond to the decrease in the condition coefficient and growth ring formation. This suggests that tilapia in Infiernillo reservoir is able to reproduce six to eight times per year, and produce the same quantity of growth rings on its hard structures.
The mean length at first maturity for O. aureus in Infiernillo is around 175 mm (Jiménez 1999), even though mature individuals from 135 mm were found. These data suggest that the rings on scales and opercular bones were due to periods of fast or slow growth imposed by reproductive energetic demands.
However, the presence of rings in hard structures from immature individuals (50 to 130 mm SL), suggest that other factors besides reproduction may determine their formation. In Infiernillo reservoir, there is a regular variation in the water level, reaching the minimum in June and the highest level in November-December. The lowest level represents a reduction of 37 to 67% of total volume of the reservoir. Around 10 to 15 km of the reservoir-bed is completely dried and used as arable land. During this time, the competition for food and space in the reservoir is presumably accentuated, leading to a reduction in growth rate of tilapia. As the rains arrive and the reservoir fills, input of nutrients from rivers and flooded cultivated lands increases primary productivity. These changes in food abundance could affect tilapia growth, and produce bands of slow and fast growth on hard structures. Payne and Collinson (1983) also suggest a similar effect of food availability and spawning on ring formation on O. niloticus scales. They suggest together with Garrod (1959) y Fagade (1973) that growth marks on hard structures in tropical fish are results of factors such as reproductive activity, feeding intensity, salinity and water level.
In a tilapia feeding study in Infiernillo reservoir, Jiménez and Nepita (2000) found no shortage of food. The diet of mature individuals was basically detritus, perhaps due to limited food resources near the nests where they moved during reproduction. Juveniles had more diverse diets, suggesting greater foraging opportunities away from reproductive areas.
So, it is proposed that ring formation on hard structures of O. aureus in Infiernillo were influenced in early stages of fish development by the reservoir dynamics, and in adults by reproduction.
Limitations and comparison between growth methods used. The deterministic nature of the von Bertalanffy model is a fundamental problem when individual variability in growth is important (Isaac 1990). The Walford (1946), Beverton and Holt (1957) and Bayley (1977) methods presented the largest deviations from observed data, due to the linearized form of the von Bertalanffy model on which they are based. So, these methods are not recommendable for populations with variable growth.
The best growth estimates were obtained when the non-linear method was used. This method makes optimised iterations of widespread combinations of L∞, K and to, so the model describes the pattern of observed data adequately. This method also provides the confidence interval for each parameter.
The method in ELEFAN package was the least adequate to evaluate tilapia growth. It is sensitive to discrete modes in the length frequency data, is not useful for multiple recruitment, and lacks a statistical error measurement (Basson et al. 1988). Multiple recruitment and indistinct modes are inherent in Tilapia populations as a consequence of multiple spawning. For these reasons, the modes in the SLCA package were masked, and provided multiple peaks in the contour surfaces, which hindered the estimate of growth parameters.
PROJMAT is based over whole monthly change in the length frequency distributions and it is therefore less sensitive to the clarity of modes (Jones et al. 1990). This method is robust even when high variation in length-age relation caused by multiple spawns and individual growth variation exists (Basson et al. 1988), such as in the present case. Therefore, this method provided a more reliable estimate of growth parameters for tilapia than the others, although to was skewed. The growth parameters estimates by FISAT package were reliable and in agreement with non-linear method estimates. So, each of the three is useful to estimate the growth in highly variable populations.
The growth parameters of O. aureus estimated by several authors for Infiernillo reservoir (Bernal 1984, Guzmán 1994, Juárez 1995 and Palacios 1995) and other reservoirs (Díaz 1985, Rodríguez 1989, Blanco 1990, Morales 1992 and Ramos 1995), in some cases showed an unrealistic growth pattern. L∞estimates have varied between 257 to 568 mm, presumably due to unrepresentative population sampling. In some cases, the growth rate is not specified as monthly or annual, or there is no reference to whether a total or standard length of the fish is used, making comparisons difficult.
Growth parameters can be variable for individuals that inhabit different reservoirs, as a function of the environmental conditions and fishing pressure to which they are subjected. In fact, environmental differences can be more important than genetics for maturation and growth of tilapias. However, the observed within-reservoir variation is likely due to nonrepresentative sampling and erroneous methodological applications.
Fishing implications on tilapia growth. In Infiernillo dam, the continuous decrease in the maximum size that O. aureus reaches by fishing effect is a worrisome aspect. Although this specie can grow up to 478 mm, nowadays the considerable represented maximum length is 275 mm standard length. These data reflect the impact that fishing has on tilapia individual growth and consequently on the population age structure, that do not surpass the year and half age. The fishing pressure since 1987, is over individuals of 11 months old that recently reached their first maturity (Jiménez 2004). It is forcing a precocious maturity of individuals, reproducing at earlier ages in order to compensate the high fishing mortality, with the consequent selection of less favourable characters of growth.
Other important change observed in tilapias is regarding to their sex proportion, possible caused by the incidence of fish near to the nest area where females take care of their broods. In 1984, Bernal reported 3 females for 1 male. Nowadays, has been a sex proportion 1 to 1 registered, which evidently reduces the number of recruits obtained for population recuperation. The degree of consanguinity in Infiernillo is considerable (Barriga et al. 2004), because all tilapia introductions have come from a reduced number of progenitors and the renovation of the stock is null, so there are a dwarfish tendency. These factors have contributed to the decrease of the population.
An appropriate regulation in tilapia exploitation in Infiernillo dam is urgent. According to Jiménez (2004), besides other recommendations, an increase in the size mesh of gill nets up to 10 cm could contribute to protect the reproductive potential of the stock, aid to recovery its population and to achieve a better yield per recruit.
Acknowledgments
This research was part of a research project development in the Regional Centre of Fishery Research in Pátzcuaro, Michoacán (CRIP-P), and was sponsored by the National Institute of Fish (INP) of México. I am grateful to Claudio Osuna and Casimiro Ramírez for their valuable help and encouragement during the field stage.
Resumen
Ante el continuo decremento en las capturas de tilapia de la presa Infiernillo, Michoacán- Guerrero, México, se estimó la edad y crecimiento de Oreochromis aureus (Steindachner 1864) que son atributos indispensables que deben conocerse para realizar el manejo de la pesquería. La evaluación incluyó la lectura de marcas de crecimiento en escamas y opérculos, y el análisis de frecuencia de tallas. También se estimó la estructura de edad de la población. Debido a la alta variabilidad individual en el crecimiento que presentan las tilapias y a fin de obtener estimaciones fidedignas de crecimiento, diferentes métodos lineales, noparamétricos y no-lineales fueron usados y su aplicación discutida. Los opérculos presentaron ventajas sobre las escamas en la determinación de edad. La reproducción y la competencia por espacio y alimento fueron consideradas factores responsables de la formación de marcas en las estructuras duras de tilapia. El modelo no-lineal fue considerado el mejor para modelar el crecimiento de poblaciones altamente variables como la de tilapia y otros peces tropicales que presentan múltiples desoves. Los parámetros de crecimiento estimados fueron: L∞ = 479 mm, K = 0.46/yr, to = -0.055 yr. La población de tilapia en la presa Infiernillo está dominada por juveniles menores de año y medio de edad. La actividad pesquera está afectando el crecimiento de la población de tilapia, puesto que éstas son capturadas antes de que desoven. La pesquería requiere urgentemente una regulación. Un incremento en la abertura de malla de las redes agalleras con que es capturada, podría contribuir a proteger el potencial reproductivo del stock.
Palabras clave: age growth models, reproductive behaviour, Oreochromis aureus, scales, opercular bones.
References
Anonymous, 1998. Diagnóstico Socioeconómico Pesquero de la presa Lic. Adolfo López Mateos, Infiernillo, para la elaboración de la propuesta de la Norma Oficial Mexicana. Oficina Regional Francisco J. Mugica. SEMARNAP. México. 69 p. [ Links ]
Anonymous, 2000. Reporte de Producción Anual de la Presa Lic. Adolfo López Mateos de 1981-1999. SEMARNAP Delegación Federal en el estado de Michoacán. Subdelegación de Pesca. Área de Informática y Estadística. México. 30 p. [ Links ]
Bagenal, T.B.& F.W. Tesch. 1978. Age and Growth, p. 98-130. In T. Bagenal (ed.). Methods for assessment of fish production in freshwater. Blackwell. IBP Handbook No. 3. London. [ Links ]
Babiker, M. & H. Ibrahim. 1979. Studies on the biology of reproduction in the Cichlid Tilapia nilotica (Linneo): gonadal maturation and fecundity. J. Fish Biol. 14: 437-448. [ Links ]
Barriga, S.I., Jiménez, B.M.L., Ibáñez, A.L. & J.L. Arredondo. 2004. Variability of tilapias (Oreochromis spp.) introduced in Mexico: morphometric, meristic and genetic characters. J. Appl. Ichthyol. 20: 7-14 p. [ Links ]
Basson, M., Rosenberg A. & J. Beddington. 1988. The accuracy and reliability of two new methods for estimating growth parameters from length-frequency data. J. Cons. Int. Explor. Mer. 44: 277-285. [ Links ]
Bayley, P. 1977. A method for finding the limits of application of the von Bertalanffy growth model and statistical estimates of the parameters. Bull. Fish. Res. Board. Can. 34: 1079-1084. [ Links ]
Bernal, B.F. 1984. Análisis de los factores relacionados con la producción pesquera de Tilapia nilotica en la Presa Adolfo López Mateos (Infiernillo), Mich.-Gro. Tesis de Licenciatura. UNAM, México, 44 p. [ Links ]
Beverton, R. & S. Holt. 1957. On the dynamics of exploited fish populations. Fisheries Investigation. Ministry of Agriculture Fisheries and Food. Sea Fisheries (Ser. 2). 19: 533 p. [ Links ]
Blanco, U.H. 1990. Algunos parámetros biológicos y pesqueros de la tilapia Oreochromis aureus Steindachner, 1864 en la presa Vicente Guerrero, Tamaulipas, México. Tesis de Licenciatura. Univ. del Noroeste, México, 54 p. [ Links ]
Castro, M. & W. Lawing. 1995. A study of sampling strategies for estimating growth parameters in fish populations. Fish. Res. 22: 59-75. [ Links ]
Díaz, L.M. 1985. Contribución al conocimiento de edad y crecimiento de Sarotherodon aureus (mojarra) de la presa "El Bosque", la Encarnación, Michoacán. Tesis de Licenciatura. UNAM, México, 50 p. [ Links ]
Eckblad, W. 1991. How many samples should be taken?. BioScience 41: 346-347. [ Links ]
Fagade, S. 1973. Age determination in Tilapia melanotherodon (Ruppell) in the Lagos Lagoon,
Lagos, Nigeria, p. 71-77. In T.B. Bagenal (ed.). The Ageing of Fish. Unwin Brothers, England.
FAO. 1982. Métodos de recolección y análisis de datos de talla y edad para la evaluación de poblaciones de peces. FAO. Circulares de Pesca. 736: 101. [ Links ]
Garrod, D. 1959. The growth of Tilapia esculenta in Lake Victoria. Hydrobiology 12 (4): 268-298. [ Links ]
Gulland, J. & S. Holt. 1959. Estimation of growth parameters for data of unequal time intervals. J. Cons. Int. Explor. Mer. 25: 47-49. [ Links ]
Gayanilo, F., Sparre P. & D. Pauly. 1993. The FiSAT Users Guide. Computerized Information Series Fisheries No. 99. FAO, ICLARM, DIFMAR Roma. 89 p. [ Links ]
Guzmán, U.A. 1994. Parámetros biológicos de tilapia (Oreochromis aureus Steindachner, 1864) de la presa Adolfo López Mateos "El Infiernillo", Michoacán- Guerrero, México. Tesis de Licenciatura, UNAM, México, 33 p. [ Links ]
Isaac, V. 1990. The accuracy of some length-based methods for fish population studies. ICLARM. Tech. Rep. 27: 1-81. [ Links ]
Jiménez, B.L. 1999. Análisis de la pesquería de tilapia Oreochromis spp. (Pisces: Cichlidae) en la presa Adolfo López Mateos, Michoacán-Guerrero. Tesis de Doctorado, Instituto de Ciencias del Mar y Limnología, México, 178 p. [ Links ]
Jiménez, B.L. & R. Nepita. 2000. Espectro trófico de la tilapia Oreochromis aureus (Perciformes, Cichlidae) en la presa Infiernillo, Mich.-Gro. México. Rev. Biol. Trop. 48: 487-494. [ Links ]
Jiménez, B.L. 2004. Application of holistic and analytical models for the management of tilapia fisheries in reservoirs. Hidrobiológica 14: 61-68 [ Links ]
Jones, J., M. Basson & S. Holden. 1990. Length Frequency Data Analysis. A prototype software package for the estimation of growth parameters from length frequency distributions. Version 2.0. Renewable Resources Assessment Group for the Overseas Development Administration. 50 p. [ Links ]
Juárez, P. 1995. Presa Infiernillo Adolfo López Mateos, p. 64-76. In E.G. De la Lanza & J.L. García (eds.). Lagos y Presas de México. Centro de Ecología y Desarrollo, México. [ Links ]
Morales, D. 1991. La Tilapia en México, biología, cultivo y pesquerías. AGT Editor. México. 190 p. [ Links ]
Morales, B.E. 1992. Evaluación de algunos aspectos biológico-pesqueros de la tilapia Oreochromis aureus (Steindachner, 1864) en la presa Vicente Guerrero (las Adjuntas) Tamaulipas. Tesis de Licenciatura, UNAM, México, 28 p. [ Links ]
Munro, J. 1982. Estimation of the parameters of the von Bertalanffy growth equation from recapture data at variable time intervals. J. Cons. Int. Explor. Mer. 40: 199-200. [ Links ]
Munro, J. & D. Pauly. 1983. A simple method for comparing growth of fishes and invertebrates. ICLARM. Fishbyte 1: 5-6. [ Links ]
Palacios, S.S. 1995. Estudio biológico pesquero de la tilapia Oreochromis aureus (Steindachner, 1864) en la presa Adolfo López Mateos (el Infiernillo), Michoacán-Guerrero, México. Tesis de Licenciatura, UNAM, México, 81 p. [ Links ]
Payne, A. & R. Collinson. 1983. A comparison of the biological characteristics of Sarotherodon niloticus (L.) with those of S. aureus (Steindachner) and other tilapia of the delta and lower Nile. Aquaculture 30: 335-351. [ Links ]
Ramos, C. 1995. Reproducción y crecimiento de la mojarra tilapia (Oreochromis aureus) en la presa Benito Juárez, Oaxaca, México. Cien. Pesquera.11: 54-61. [ Links ]
Ricker, W. 1975. Computation and Interpretation of Biological Statistics of Fish Populations. Bull. Fish. Res. Board. Can.191: 203-211. [ Links ]
Rodríguez, P.A. 1989. Evaluación de las pesquerías de tilapia, carpa, bagre y charal en el Lago de Chapala, Jalisco-Michoacán en el período de julio de 1987 a julio de 1988. Tesis de Licenciatura, UNAM, México, 130 p. [ Links ]
Saila, B., H. Prager & W. Recksiek. 1988. Basic Fishery Science Programs: a compendium of microcomputer programs and manual of operation. Elsevier Scientific, Bronxville, New York. [ Links ]
Sparre, P. & S. Venema. 1995. Introduction to tropical fish stock assessment. Part. I. Manual FAO Fish. Tech. Paper. 306: 15-64. [ Links ]
Walford, L. 1946. A new graphic method of describing the growth of animals. Biol. Bulletin of Marine Biology Laboratory Woods Hole. 90: 141-147. [ Links ]
Wolff, M.1989. A proposed method for standardization of the selection of class intervals for length frequency analysis. Fishbyte 7: 5-6. [ Links ]
Zar, H.J. 1974. Biostatistical Analysis. Prentice-Hall Biological Sciences Series, USA. 620 p. [ Links ]